Springs – oscillations

Question:

I am doing some physics coursework and cannot find what I'm looking for. My coursework title is "How does the mass on the end of a spring affect the time period of the spring?" We put different masses on the end of a spring and then extended the spring an extra 2 cm (to increase the tension apparently). We then let it go and timed how long 10 oscillations of the spring took, we divided it by 10 to get the time period of 1 oscillation, we then repeated this with other masses being put on the end of a spring. I have lots of notes which I just don't understand!

I would be really grateful for any help you could give me. I cannot seem to find anyone else who is doing the same coursework either, the coursework needs to be handed in soon too! I have been trying for a long time to understand it.

Answer:

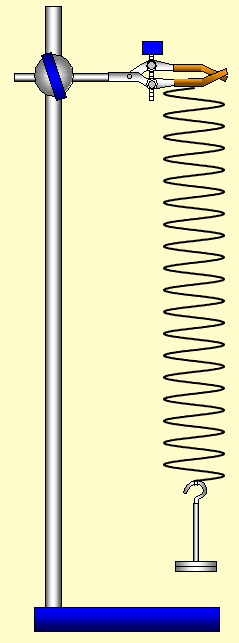
1. Suspend your spring from a support.
2. Put an initial load on the spring – say 100g
3. Pull the spring down a little way (about 5 cm although the actual distance does not matter) and then let go.
4. Time 10 oscillations (you can start at any time but best at either the top or bottom of an oscillation.
5. Divide by ten to get the time for one oscillation.
6. Add another 100 g (50g if you can) and repeat the procedure for another five or six masses. You really need six to get a reliable graph, eight is even better. It is not necessary to pull the mass down the same distance each time because the period (T) does not depend on the amplitude as long as the amplitude is not too big.
7. Make a table of the mass and the time for one oscillation
8. Plot a graph of mass (M) (y axis) against time (T) (x axis)
This should give you a curve, the T values increasing faster than the M values.
9. Plot a second graph of (M) (y axis) against T squared (x axis)
This should give you a straight line.
N.B You must not get the spring to extend beyond its elastic limit. The way to check this is to make sure that the spring goes back to its original length after each load.

schoolphysics oscillating spring animation

To see an animation of the motion of an oscillating spring click on the animation link.

Theory of the oscillation of a mass on a helical spring

The theory of this is really for students in the 16-19 age range but I will give it you all the same. Most textbooks for this age group have it and it is also on the web site. Don't worry if you do not follow it yet.


Consider a mass m suspended at rest from a spiral spring and let the extension produced be e. If the spring constant is k we have: mg = ke
The mass is then pulled down a small distance x and released.
The mass will oscillate due to both the effect of the gravitational attraction (mg) and the varying force in the spring (k(e + x)).
At any point distance x from the midpoint: restoring force = k(e + x) - mg
But F = ma, so ma = - kx and this shows that the acceleration is directly proportional to the displacement, the equation for simple harmonic motion.
The negative sign shows that the acceleration acts in the opposite direction to increasing x.

From the defining equation for simple harmonic motion. (a = - ω² x) we have ω =k/m=g/e and therefore the period of the motion T is given by:
Period of oscillation of a helical spring (T) = 2π√(m/k).